The single photon is one of the most abundant and ubiquitous of elementary particles. On any given day, we perceive trillions upon trillions of visible optical photons, we feel the warmth of quintillions of infrared thermal photons, and countless gamma photons pass through us. Yet as individual particles, single photons are difficult to detect.
Single photons are often the only detectable energy signature in so-called photon-starved applications such as astronomy, long-range laser radar imaging, DNA fluorescence, and sensing or imaging through absorbing media (e.g., biomedical tissue and through-body examination). While techniques exist to detect single photons and other elementary particles, many of them require bulky equipment, are inefficient, and lack the bandwidth and capability for wide-area sensing and imaging. There is an ongoing push to develop single photon sensor arrays that can count single photons and use the counted single photons to construct an image of the target object. So far, the grand aim of producing high-resolution single-photon sensors is not yet attainable.
In this article we will review the various single photon counting techniques, discuss linear avalanche photodiode (APD) detectors—a new genre of single photon counters—and demonstrate that they have promising single photon sensor performance characteristics and applications.
Single Photon Instrumentation
The block diagram of a typical single photon counting instrumentation is shown in Figure 1. The single photon generates charge carriers in the optical detector, which is the most important component in the single photon sensor. The photocurrent caused by these charge carriers is amplified by a broadband low-noise amplifier. The output of the amplifier is a voltage pulse that can typically have an irregular shape. Once a portion of this pulse exceeds a threshold voltage, it is usually assumed a single photon has been detected. The shaper/discriminator performs the role of a threshold detector, which outputs a well-defined pulse that is registered by the counter. The amplifier, discriminator/shaper, and counter can be any of a number of commercially available components, selected on the basis of high bandwidth and minimal noise performance.
 Figure 1. Block diagram of single photon counting instrumentation |
Single Photon Detectors
Single photon counting detectors fall into four broad categories: vacuum detectors; semiconductor detectors; linear-mode detectors; and Geiger-mode detectors. They all respond to incident photons by generating photocurrents and multiplying the photogenerated charges. Charge multiplication or photoelectric gain is a critical requirement for single photon counting detectors because of the high background and detector noise. The photocurrent is related to the incident optical power by Equation 1:
| (1) |
where:
| Iph | = | photocurrent |
| λ | = | photon wavelength |
| c | = | photon velocity |
| h | = | Planck's constant |
| q | = | electronic charge |
| Pin | = | incident optical power (W) |
| M | = | photoelectric gain |
| η | = | quantum efficiency |
M, the photoelectric gain, can be due to multiplication in a vacuum tube or in a semiconductor layer specially designed to accelerate photogenerated charge carriers so that they collide with other charge carriers and generate an avalanche of charges. M should be as high as possible to maximize the photocurrent. The quantum efficiency is a measure of the photon's ability to generate charge carriers without any charge multiplication. Ideally, good detector materials and devices should convert 100% of incident photons into charge carriers.
The dark current is the current that flows without any incident photon and it depends on both the applied voltage and the quality of the detector material. It is responsible, along with background noise and signal noise from the incident photons, for the detector noise that determines the ultimate sensitivity of the detector. In general, most detectors are dark current limited. The key requirements for the highest performing single photon detector are high quantum efficiency, high photoelectric gain, and low noise current. Since the optical gain process also produces noise currents, the photoelectric gain cannot be indiscriminately increased. Optimizing the detector's performance requires a trade-off between the detector gain and the noise.
Because a single photon cannot be detected with the highest level of certainty (due to its statistical nature as an elementary particle) the performance of a single photon counting detector is usually expressed in terms of its single photon detection probability. This parameter is governed by Poisson statistics and is a function of the photocurrent and noise current. The probability of single photon detection is usually less than 100%.
Figure 2 summarizes the performance parameters for current single photon detector technologies. Vacuum detectors include photomultiplier tubes (PMTs) and intensified photodiodes which are actually PMTs with semiconductor cathodes. Vacuum detectors usually have very high photoelectric gains and low quantum efficiencies. They require very high-voltage power supplies and they cannot be easily implemented as arrays. Semiconductor detectors for single photon counting are usually based on avalanche photodiodes (APD) of silicon (Si), indium-gallium-arsenide (InGaAs) or mercury-cadmium-telluride (HgCdTe or MCT). Their photoelectric gain is usually lower than that of vacuum detectors, but the quantum efficiencies are much higher. The key attributes of semiconductor detectors are their lower power supply requirements and the potential to fabricate large-format, high-resolution arrays with them.
Figure 2. Performance comparison of current single photon detectors
| PMT | Geiger-mode APD | Linear-mode APD | |
| Photoelectric gain below breakdown | 10,000–1,000,000 | 10–100 | 10,000–300,000 |
| Excess noise factor | <2 | >2 | 2 |
| Max. detection probability | <25% | <50% | 25%–100% |
| Operating temperature | 240°K | 240°K | 300°K |
| Voltage bias | >1000 V | 30–70 V | 30–40 V |
| Detection speed | <1 ns pulses | >20 ns pulses | <1 ns pulses |
| Afterpulsing | No | Yes | No |
| Wavelength | ≤1.6 µm | 1–2 µm | ≤2 µm |
| Magnetic field susceptibility | Yes | No | No |
| Reliability | <1000 hr. | <100,000 hr | >1000 hr. |
| Large-format array capability | No | Yes | Yes |
| Crosstalk | N/A | Poor | No |
Because of their high photoelectric gain, PMTs and other vacuum detectors can be operated in the linear mode, in which each incoming single photon generates a precise detector output pulse, two single photons generate double this output pulse, and so on and so forth with multiple photons. This is the most desirable type of single photon counting and also enables photon number analysis. However, the low quantum efficiency of PMTs means that the single photon counting probability of these devices is still lower than desired.
In contrast, semiconductor detectors have high photoelectric gain only above the photodiode breakdown voltage. In this operating regime, each single photon generates photocurrent. However, because of the avalanche mechanism that produces the extra photocurrent (due to the gain), it also produces substantial noise current. This technique is referred to as Geiger mode because it is similar to the gas discharge pulse in a Geiger tube (commonly used to detect single particles of ionizing radiation). However, Geiger-mode photon counting is a digital, nonlinear detection approach and cannot resolve multiple photons. Further, Geiger-mode detectors require low temperatures to achieve reasonable single photon detection probabilities. They also suffer from afterpulsing, which prevents the attainment of low pulse-widths, allows only one measurement in a pulse cycle, and limits the maximum achievable pulse repetition rate and bandwidth. To date, single photon counting APDs are capable of operation only in the Geiger mode.
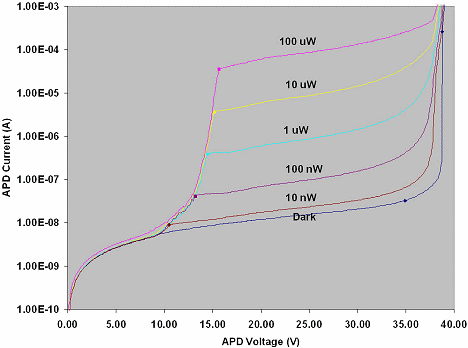
Recently, linear-mode APDs—which have the best features of PMTs without their drawbacks—have been developed (see sidebar "Linear-mode Single Photon APD"). In contrast to Geiger-mode APDs, linear-mode APDs can operate below the breakdown voltage with high gain and low noise to enable high single photon counting performance. The high gain is achieved in one of two ways. The first is by combining optical gain with APD avalanche gain, as occurs with the optically preamplified APD detectors, which integrate an optical amplifier with the detector. The second method uses a detector designed to have exceedingly high gain without optical amplification. In the former case, both optical amplifier and APD can each have gains not exceeding 100 for a total detector gain in excess of 10,000: a distinct advantage which minimizes both amplifier and detector noise and enables the attainment of detection probabilities approaching 100%. For the linear APD without optical amplification, the device is designed to have a linear photoresponse from picowatt power levels to milliwatts as the light-current-voltage characteristics in Figure 7 in the sidebar show. Recently, an APD design has been introduced that suppresses noise generated by multiplication due to positive charges and has been used to achieve simultaneous photoelectric gains ranging from 10,000 to >100,000 with low excess noise.
Single Photon Detection Probability
The basic requirement for a single photon counting instrument is that the photoelectric gain should be sufficiently high to overcome any signal due to noise electrons introduced by the detector and associated electronic components. Using detection theory, the photon counting probability can be expressed as an inverse exponential function of the threshold-to-noise ratio (TNR) and the signal-to-noise ratio (SNR). Since both SNR and TNR are directly related to the photoelectric gain and the noise threshold in terms of the photoelectrons and noise electrons, respectively, the detection probability (p) is given by Equation 2:
| (2) |
where:
| g | = | gain in signal photoelectrons |
| σ | = | noise electron threshold above which detection occurs |
The gain in signal photoelectrons can be determined from Equation 1 in terms of the quantum efficiency and the photoelectric gain. A noise model to evaluate the noise threshold for a single photon counting system is shown in Figure 3. The principal noise sources are dark current in the detector, excess noise from the detector due to the photoelectric gain processes, and Johnson noise from the downstream electronics such as the transimpedance amplifier (TIA) and discriminator. The detector noise electrons are given by Equation 3 :
| (3) |
where:
| n | = | number of detector noise electrons |
| τ | = | pulse duration |
| q | = | electronic charge |
| Id | = | dark current |
| Be | = | electronic bandwidth |
| M2.7 | = | terms for the detector excess noise |
To simplify the analysis, we assume that the pulse-width of the optical signal is 1 ns, and the electronic bandwidth is 1.4 GHz. Also, we assume the Johnson noise current for the downstream electronics (such as the TIA and discriminator) is ~100 nA, which is equivalent to 625 noise electrons for a 1 ns duration.
The photon counting probability is estimated using this noise model, Equation 2, and actual data for typical Geiger-mode and linear APDs. The estimated performance of two single photon counting detectors is illustrated in Figure 4. The calculations assume APDs with low k-factor and low dark current (32 nA). The detectors designed for Geiger-mode operation have lower gain and are capable only of low detection probabilities. Linear-mode APDs, on the other hand, can attain both higher gains and higher single photon detection probabilities. Additional analysis shows that, with lower dark currents, the linear APD can attain single photon detection probabilities approaching 100%.
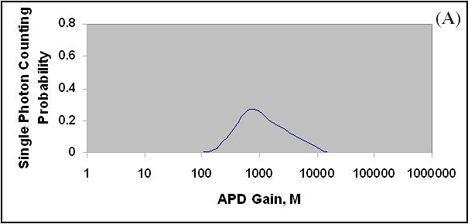  Figure 4. Simulated single photon counting probability for Geiger- and linear-mode APD (images A and B, respectively) |
Applications
Single photon sensors are most needed in the so-called "photon-starved" applications in which a single photon is the end result of optical propagation through a medium under observation. Such applications include long-range laser radar (ladar), astronomy, through-body biomedical imaging, DNA sequencing, picosecond spectroscopy, and quantum encryption and computing.
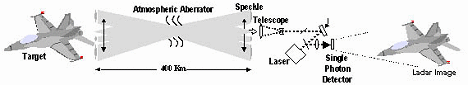
An example of a ladar application (Figure 5) involves imaging of a target located several hundred kilometers away. A high-energy, pulsed laser signal is directed at the target. The transmitted and reflected signal is highly attenuated by the propagation process, atmospheric scattering, and atmospheric aberrations, all of which combine to reduce the intensity of the return signal to little more than that of a single photon. In other words, of all the 1018 photons transmitted in a typical pulse cycle for such a laser, only a single photon is reflected back to be detected and used for distance determination and imaging. For high resolution imaging, the single photon sensor must have a probability of detection greater than 70% and must be capable of picosecond time resolution.
Applications for sensing, spectroscopy, and imaging of biomedical specimens include so-called through-body molecular imaging where only single photon signals are transmitted through a biological specimen that has severely degraded the original optical signal. DNA sequencing, flow cytometry, and confocal microscopy also require single photon sensing, because extremely weak fluorescence emissions need to be detected at the single photon level. In these applications, existing single photon detectors lack the required detection probabilities, freedom from timing jitter, and picosecond pulse response.
Encrypted secure communication and quantum computing employ the properties of single photons as an elementary particle. These applications include quantum key distribution (QKD) in which single photons with differing polarizations or phases are used to represent digital bits of "1" and "0." QKD is implemented by transmitting single photons through a potentially insecure fiber-optic communication line. Using the single photon bits as the encryption key, this telecommunication approach is completely secure and impervious to intercept without the knowledge of the sender and receiver. Prototype QKD secure communication terminals have recently been introduced into the market. These QKD systems are limited by the communication range, data rate, and bit error rate caused by the limitations of currently available single photon detectors.
Looking Forward
With the development of semiconductor-based single photon counting sensor arrays, single photon sensing can now be transitioned from the benchtop to a wide variety of field applications in laser radar, biomedical imaging, and telecommunication systems. Of all the single-photon counting techniques, the linear APD detector has shown the greatest potential for high detection probability, speed, and large-format array production. Its full development will usher in a new era of sensing and imaging with this widely familiar elementary particle.
Further Reading
1. R.H. Kingston, Detection of Optical and Infrared Radiation, Springer Verlag Heidelberg, New York 1978
2. Robert Nigel Tubbs, Lucky Exposures: Diffraction Limited Astronomical Imaging Through the Atmosphere, Doctoral Dissertation, St. Johns College, Cambridge University, Cambridge, UK, September 2003
3. Leye Aina, Ayub Fathimulla, Harry Hier, Mark Lecates, Parth Patel, Sachi Babu, Jim Foshee, "Non-Geiger-mode single photon counting APDs with high detection probability & afterpulse-free performance," SPIE Optics East Conference, Boston, MA, September 9, 2007
4. Leye Aina et. al., "Linear-mode single photon counting APD arrays with subnanosecond, afterpulse-free performance for ladar, spectroscopy & QKD applications", Proc. of SPIE, Vol. 6572, 65720H, (2007)
5. Joe C. Campbell et al., "Recent Advances in Avalanche Photodiodes", IEEE Journal of Selected Topics in Quantum Electronics, Vol. 10, no. 4, July/August 2004
6. Xaoli Sun & Frederic Davidson, "Photon Counting with Silicon Avalanche Photodiodes," Journal of Lightwave Technology, Vol. 10, p. 1023, August 1992.
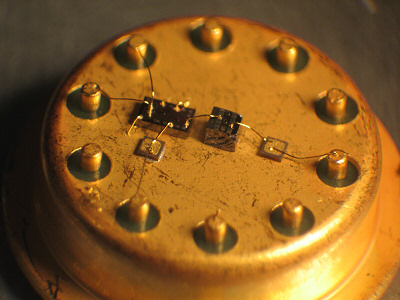
| Linear-Mode Single Photon APD The single photon counter illustrated in Figure 6 has optical gain in excess of 100,000 and low dark current to enable linear-mode single photon counting that can numerically distinguish between single, double, and multiple photons (Figure 7).
|
This article was originally presented at the SPIE Optics East conference held in Boston, MA, in September 2007 and the SPI Defense & Security Conference held in Orlando, FL, in April 2007.