|
Force Per Unit Area
In a fluid, the pressure at a given point is independent of direction. It can be considered a compression stress. Without flow, shearing stresses cannot exist. As such, no component of stress can exist along a solid boundary, in a static fluid, or along an arbitrary section passed through the fluid. This means that pressures must be transmitted to solid boundaries or across arbitrary sections normal to these boundaries or sections at every point. Because fluids are continuous media, pressure imposed on a fluid at rest is transmitted undiminished to all other points in the fluid and to the walls of the containing vessel (Pascal's law).
An understanding of Pascal's law is fundamental to understanding pressure and pressure measurement. The following illustration will help you understand this concept.
In a hydraulic jack, you apply force (F1) to a piston of area (A1). The resulting pressure (F1/A1) applied to the fluid is transmitted throughout the connected volume of fluid. This occurs because fluids can't sustain shear strain: different parts of the fluid can't be at different stress (pressure) levels. Fluids have a low modulus in shear (what enables them to flow).
Because the pressure stress is equal throughout the volume of the fluid, the fluid exerts equal pressure on all parts of its container. So, pressure F1/A1 is exerted equally on all points of the structure of the hydraulic jack fluid system. Exerting pressure F1/A1 on area A2 results in a total force (F2) on A2. F2 = (F1/A1) × A2.
Thus, the small force applied on piston 1, area A1, results in a large force on piston 2, area A2. Because nature seldom gives you something for nothing, you sacrifice distance traveled (stroke length) to get higher force—the volume of fluid moved by piston A1 is the same as the volume displaced by piston A2. Because area A2 is greater, the same volume results in a shorter displacement. Of course, in a real hydraulic jack, you have a reservoir of fluid and a system of check valves, which let you repeat the stroke of piston A1 without losing the displacement of A2.
Pressure Generated by Weight
The force required to generate pressure can be provided by brute force, as with the hydraulic jack, or it can be provided by gravitational acceleration on a mass. The gravitational force is commonly called weight. So, rather than pushing down on piston A1, you can place weight on it. Unfortunately, you may easily become entangled in a confusion of units of weight, force, mass, and acceleration.
Weight is the force exerted by gravity on a mass. However, in U.S. units, you normally assume standard gravitational acceleration and ignore mass. Thus, a 10 lb. weight exerts a force of 10 lb. on earth's normal gravitational field, and you have no good way of expressing mass. Because gravity varies only a few percent over most of the earth's surface, you create a possible error of a few percent.
In metric units, force, mass, and acceleration are not related to earth's gravity, but weight is. If you quantify the system in terms of mass, you must convert to force (weight) caused by earth's gravity.
Assuming that Newton was correct, and F = ma, then:
W = mg
where:
| F | = force |
| m | = mass |
| a | = acceleration |
| W | = weight |
| g | = local acceleration of gravity |
Because of the variety of units used for weight, mass, and acceleration, these equations must be modified to include a conversion constant b:
F = bma and W = bmg
where:
| b | = a numerical factor to ensure consistency of units |
| b | = 1 W in newtons, m in kilograms, g in m/s2 |
| b | = 1 W in dynes, m in grams, g in cm/s2 |
| b | = 1 W in poundals, m in pounds-mass, g in ft./s2 |
| b | = 1 W in pounds-force, m in slugs, g in ft./s2 |
| b | = 1 W in pounds-force, m in pounds-mass, g in gravity |
| b | = 1/32.17 405 W in pounds-force, m in pounds-mass, g in ft./s2 |
| b | = 1/980.665 W in pounds-force, m in pounds-mass, g in cm/s2 |
| b | = 1/9.80 665 W in kilograms-force, m in kilograms-mass, g in m/s2 |
By international agreement, the standard gravity unit acceleration is 9.80665 m/s2. The U.S. Coast and Geodetic Survey indicates that g varies less than ±0.3% over the continental U.S. and less than ±3% in industrialized cities of the world.
|
Pressure of a Fluid Column
The force that generates pressure can be caused by any weight or mass. Therefore, the weight of the fluids' mass generates pressure. A column of fluid generates pressure proportional to the density of the fluid and the vertical height of the column. The pressure at a given depth is independent of the area of the column and the shape of the container (see Figure 1).
The weight (W) of the fluid in the container is distributed over the area (A) of the base. At the bottom of the container:
P = W/A;
because W = bmg and P = bmg/A
Mass (m) = density (r) × volume (V),
so P = brVg/A
V = Ah,
so P = brAhg/A, and
P = brgh
Thus, P is independent of A and dependent only on b (unit conversion constant), r (volumetric density, weight per unit volume), h (height of column), and g (acceleration of gravity). So, if you ignore the slight local variations of gravity and have consistent units, P = rh.
It should also be apparent that the pressure measured at any depth in the column is proportional to depth. This phenomenon is used for measuring pressure head. For example, the pressure 100 in. below the surface of a column of mercury at 80°F (density = 0.48879 lb./in.3) is P = rh = 0.48879h. At a depth of 100 in., P = rh = (0.48879) (100) = 48.879 psi. At 50 in., P = rh = (0.48879)(50) = 24.4395 psi.
If the surface of the mercury is exposed to local atmospheric pressure, it will be pounds-per-square-inch gauge. If it's exposed to a vacuum, it's pounds-per-square-inch absolute.
A critical variable for accurate conversion from head pressure to pounds per square inch is the temperature of the fluid because density varies with temperature. With large head, the head pressure can also slightly increase density near the bottom of the column. Most liquids are essentially incompressible, so this is usually insignificant. However, if the fluid is a compressible gas (e.g., the atmosphere), the compressibility can be significant. Thus, atmospheric pressure doesn't vary directly with altitude (depth), but water pressure is nearly directly proportional to depth.
Force Generated by Pressure
For the walls of a fluid container to remain stationary, the force exerted by the pressurized medium must be opposed by an equal and opposite force. Imagine what happens when the fluid pressure force is greater than the opposing force—the vessel ruptures or the piston moves, a much more desirable result. Summing all the forces caused by the fluid pressure on all the infinitesimal areas of the piston, you find that the total force is equal to the pressure times the area:
F2 = (F1/A) × A2 = P × A2
And this is true for any area of the container you choose.
Dynamic Effects
Thus far, when you've applied a force or measured the effect of a force, you've done so under steady-state conditions—after making the change (applying a transient), you let the system reach a long-term equilibrium state, where any further changes are too slow to be of any interest. These are called static pressure measurements, or steady-state measurements. This technique is useful in applications in which you're only interested in equilibrium conditions or in slowly changing conditions.
But you're often interested in monitoring the rate of change, a pattern of change, a trend, or small changes of pressure over short intervals of time. These are called dynamic pressure measurements.
Dynamic pressure measurements present more problems than do static pressure measurements. Not only do you have to consider the frequency response of the measurement system, but you must also consider the effects of the container and any connecting hardware. And by definition, you can't wait for equilibrium conditions.
Pressure Units and Conversions
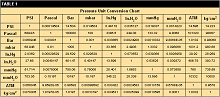 Click the image above for Pressure Unit Conversion Chart |
Following are some definitions of pressure terminology, types of pressure, and different ways of measuring pressure (see Figure 2).
 Figure 2. Pressure is always measured relative to some reference. If the reference is absolute vacuum, the pressure is absolute pressure. If the reference is local ambient pressure, the pressure is gauge pressure. If you're measuring a pressure difference between two points without regard to the absolute or gauge pressure, the measurement is differential pressure. |
Pressure head is the height of a liquid column at the base of which a given pressure would be developed due to gravity acting on the fluid mass.
Differential pressure is the difference in pressure between two points of measurement. If the pressure at one point in a system is 150 psig and at another point is 75 psig, the differential pressure is 75 psid. The reference is usually the lower pressure.
Common mode pressure is the pressure common to both ports of a differential pressure measurement system. In the previous example, common mode pressure is 75 psig.
Atmospheric pressure (barometric pressure) is the pressure caused by the weight of the earth's atmosphere. Barometric pressure varies with geographic location, altitude, and weather. It can also be affected by air conditioning systems and cleanroom enclosures.
Standard pressure is a pressure of one normal (standard) atmosphere (defined in the U.S., but some industries and other countries use a different standard), such as:
101325 Pa
101.325 kPa
1013.25 mbars
14.696 psia
29.921 in.Hg @ 0°C (32°F)
760 mmHg @ 0°C (32°F)
407.5 in.H2O @ 20°C (68°F)
33.958 ft.water @ 20°C (68°F)
Gauge pressure is measured relative to the local ambient pressure. It's the difference between the measured pressure and atmospheric pressure, unless the ambient pressure surrounding the sensor is different from the atmospheric pressure.
Sealed gauge pressure is measured with reference to the pressure in a sealed container. The container is usually located within the sensor. If the sealed reference is near absolute vacuum, this would be absolute pressure.
Vacuum is pressure measured below atmospheric pressure, referenced to atmospheric pressure. Vacuum is negative gauge pressure. Because gauge pressure is measured relative to local atmospheric pressure, it can be either positive or negative. Perfect vacuum is zero absolute pressure and indicates the complete absence of any matter (see Figure 3).
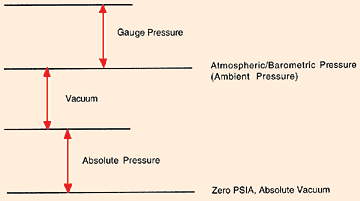 Figure 3. Pressure less than atmospheric pressure can be measured relative to atmospheric (i.e., a negative gauge pressure) and is called vacuum. Pressure less than atmospheric pressure can also be measured relative to absolute vacuum as absolute pressure. |
Absolute pressure is measured relative to zero pressure or a perfect vacuum. It's gauge pressure plus ambient pressure. Some sensors measure absolute pressure by measuring pressure relative to a vacuum sealed in a reference chamber in the sensor. The zero offset of the sensor can be used to adjust a gauge or sealed gauge pressure reading to provide an indication of absolute pressure.
Acknowledgment
Much of the material and illustrations in this tutorial is derived from the textbook Dynamic Pressure Measurement Technology, which was edited by Jon Wilson and published by the Endevco Division of Meggitt Corp.

